Hardy-Weinberg equilibrium

Hardy-Weinberg equilibrium (HWE) is a fundamental concept in population genetics that describes the genetic variation in a population under certain conditions. It provides a mathematical model to study the genetic makeup of a population and to understand the forces that can cause changes in allele frequencies over time.

What is Hardy-Weinberg equilibrium ?

Hardy-Weinberg equilibrium (HWE) is a fundamental notion in population genetics that explains a population’s genetic makeup in the absence of evolution. It states that in the absence of evolutionary forces, allele and genotype frequencies in a population will be stable from generation to generation. This equilibrium serves as a baseline against which to compare observed genetic data, allowing researchers to determine whether and how populations evolve.

Conditions of Hardy-Weinberg Equilibrium

To be in Hardy-Weinberg equilibrium, a population must satisfy the following five conditions:

• Large Population Size: The population must be sufficiently enough to reduce random changes in allele frequencies (genetic drift).
• No Mutation: There are no mutations that affect allele frequencies.
• No Migration: Individuals do not move into or out of the population.
• Individuals in the population mate randomly based on their genetics.
• No Natural Selection: All genotypes have equal probability of survival and reproduction, implying that no selective pressures favor specific alleles.

Hardy-Weinberg Principle

The principle uses two primary alleles at a single gene locus to predict the frequency of genotypes. Let’s denote:

• p as the frequency of one allele (A).
• q as the frequency of the other allele (a).

Since there are only two alleles, their frequencies sum to 1:

p+q=1

The expected genotype frequencies in the population can be calculated using:

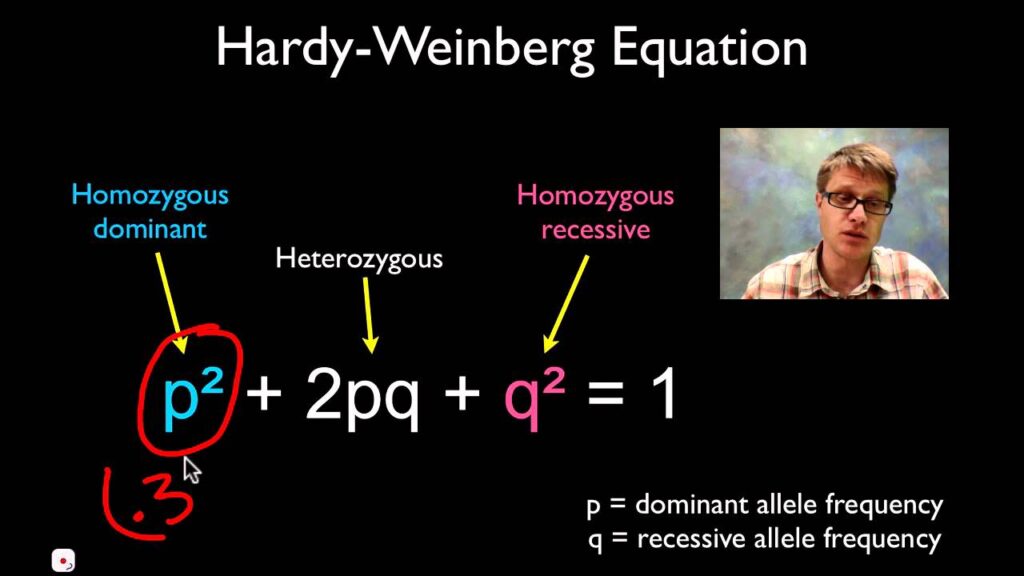
• p² for the frequency of the homozygous dominant genotype (AA).
• 2pq for the frequency of the heterozygous genotype (Aa).
• q²for the frequency of the homozygous recessive genotype (aa).

Therefore, the equation representing the equilibrium is: p²+2pq+q²=1

Example Calculation

Imagine a population where the frequency of allele A (p) is 0.6 and the frequency of allele a (q) is 0.4.

1. Calculate the expected genotype frequencies:

Frequency of AA: p²=0.6²=0.36

Frequency of Aa: 2pq=2×0.6×0.4=0.48

Frequency of aa: q²=0.4²=0.16

2. Check the sum of the frequencies:

0.36+0.48+0.16=10.36 + 0.48 + 0.16 = 10.36+0.48+0.16=1

Deviations from the Hardy-Weinberg equilibrium

When observed genetic data differs from Hardy-Weinberg expectations, it indicates that one or more of the equilibrium requirements are not met. Possible sources of variances are:

• Genetic drift: Random changes in allele frequencies can occur, particularly in small populations.
• Mutation introduces new alleles, which alter allele frequencies.
• Gene flow occurs when individuals migrate into or out of the population, introducing new alleles or removing existing ones.
• Non-random Mating: If people prefer certain mates based on their genotype, this can affect genotype frequencies.
• Natural selection: Different genotypes’ survival and reproduction rates influence allele frequencies.

Applications of Hardy-Weinberg Equilibrium

• Population Genetics Studies: Offers a theoretical framework for investigating genetic variation and identifying evolutionary causes.
• Evolutionary Biology: Examines changes in allele frequencies to better understand evolution’s causes.
• Forensic Science: Calculates the likelihood of genetic profiles in a population, which aids criminal investigations and identity verification.
• Conservation biology involves monitoring genetic diversity within endangered species populations and developing conservation measures.

Frequently Asked Question

Related Article

Epigenetics and its roles in plants biotechnology